LaPlace Transform

Colorized Definition

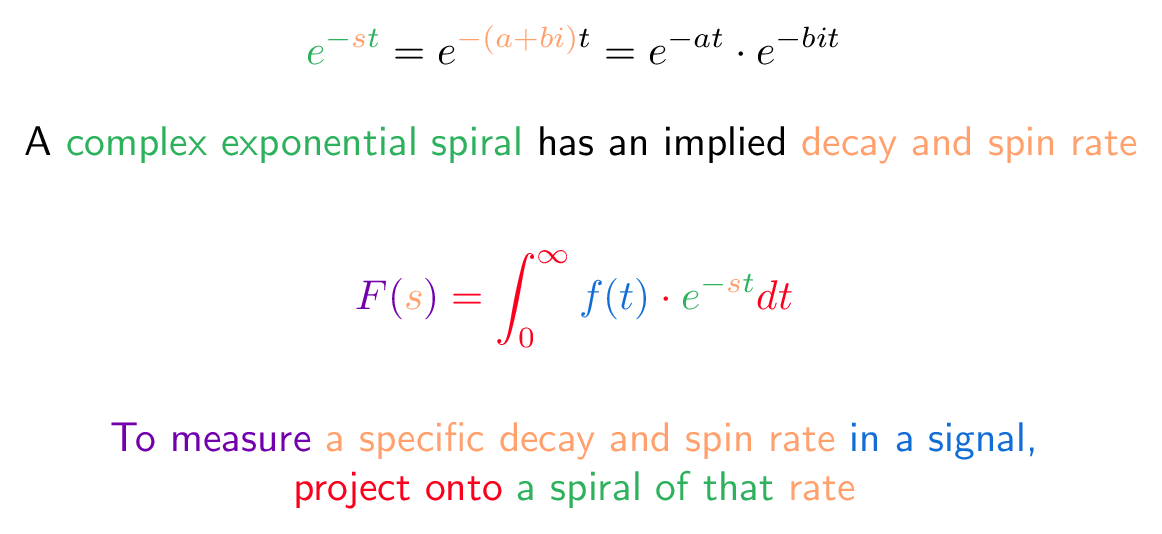
\newcommand{\energy}{\color{c1}}
\newcommand{\spiral}{\color{c2}}
\newcommand{\project}{\color{c3}}
\newcommand{\signal}{\color{c4}}
\newcommand{\rate}{\color{c5}}
\newcommand{\alltime}{\color{c6}}

$$
\spiral e^{-
\rate   s
\spiral t}
\plain = e^{
\rate -(a+bi)
\plain t} = e^{-at} \cdot e^{-bit}
$$
\plain   A
\spiral  complex exponential spiral
\plain   has an implied
\rate    decay and spin rate

$$
\energy F(
\rate s
\energy )
\project = \int_0^\infty
\signal f(t)
\project \cdot
\spiral e^{-
\rate s
\spiral t}
\project dt
$$

\energy    To measure
\rate      a specific decay and spin rate
\signal    in a  signal,
\\
\project   project onto
\spiral    a spiral of that
\rate      rate