BetterExplained Wiki

# Bayes' Theorem

# Colorized Definition

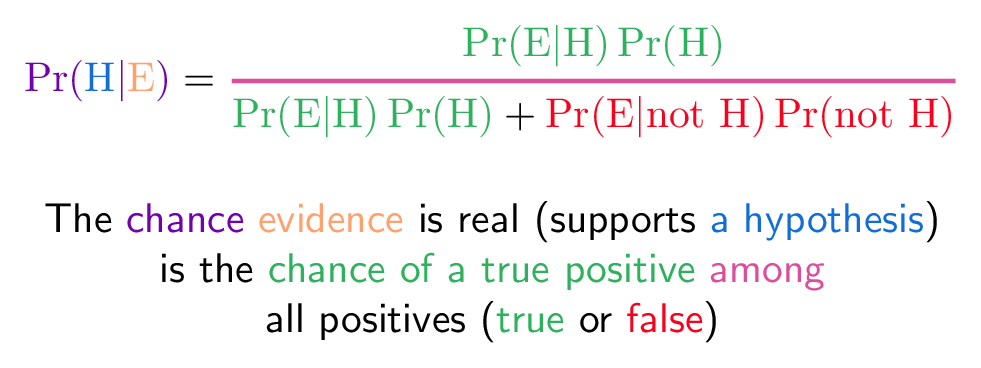
Bayes' Theorem

\newcommand{\chance}{\color{c1}}
\newcommand{\truepos}{\color{c2}}  % green (true)
\newcommand{\falsepos}{\color{c3}} % red (false)
\newcommand{\hypothesis}{\color{c4}}
\newcommand{\evidence}{\color{c5}}
\newcommand{\among}{\color{c6}}

$$
\chance \Pr(\mathrm{ \hypothesis H}|\mathrm{ \evidence E})
\plain =
\among \Frac{
  \truepos \Pr(\mathrm{E}|\mathrm{H})\Pr(\mathrm{H})
}{
 \truepos \Pr(\mathrm{E|H})\Pr(\mathrm{H})
\plain +
\falsepos \Pr(\mathrm{E | not \ H})\Pr(\mathrm{not \ H})}
$$

\plain      The
\chance     chance
\evidence   evidence 
\plain      is real (supports
\hypothesis a hypothesis\plain) \\ is the
\truepos    chance of a true positive
\among      among \\
\plain      all positives (\truepos true \plain or \falsepos false\plain)

# Read More

Combination →